Úlohy: 1–20 / 183

12345
Přehled ročníků

1. Parametrické a obecné rovnice

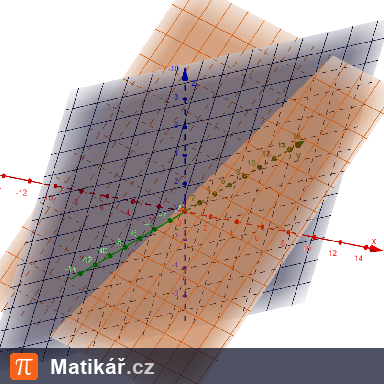
Jsou dány parametrické rovnice.

Převeďte rovnice na obecný tvar:
a)   \( x = 2t + 1, \quad y = 3t - 4 \)
b)   \( x = t - 3, \quad y = 4t + 2 \)
c)   \( x = 5t + 2, \quad y = 6t - 1 \)
d)   \( x = -2t + 4, \quad y = t - 5 \)
e)   \( x = 3t + 7, \quad y = -t + 2 \)
f)   \( x = 4t - 6, \quad y = 2t + 8 \)
Řešení
a)   \( 3x - 2y - 11 = 0 \)
b)   \( 4x - y + 14 = 0 \)
c)   \( 6x - 5y - 17 = 0 \)
d)   \( x + 2y + 6 = 0 \)
e)   \( x + 3y - 13 = 0 \)
f)   \( 2x - 4y + 44 = 0 \)

2. Obecné a parametrické rovnice

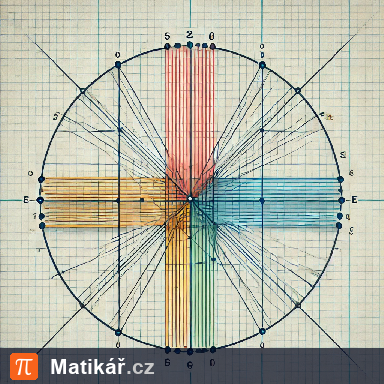
Jsou zadané obecné rovnice přímek v rovině.

Převeďte rovnice na parametrický tvar:
a)   \( 2x + 3y - 6 = 0 \)
b)   \( x - 4y + 8 = 0 \)
c)   \( 3x + 2y - 12 = 0 \)
d)   \( 5x - y - 15 = 0 \)
e)   \( x + y - 7 = 0 \)
f)   \( 4x - 3y + 9 = 0 \)
Řešení
a)   \(x = t, \quad y = \frac{6 - 2t}{3}\)
b)   \(x = t, \quad y = \frac{t + 8}{4}\)
c)   \(x = t, \quad y = \frac{12 - 3t}{2}\)
d)   \(x = t, \quad y = 5t - 15\)
e)   \(x = t, \quad y = 7 - t\)
f)   \(x = t, \quad y = \frac{4t + 9}{3}\)

3. Kvadratické nerovnice

Vyřešte kvadratické nerovnice:
a)   \(x^2 - 4x - 5 > 0\)
b)   \(x^2 + 3x - 10 \leq 0\)
c)   \(2x^2 - 8x \geq 0\)
d)   \(-x^2 + 5x + 14 < 0\)
e)   \(x^2 - 6x + 9 > 0\)
f)   \(2x^2 - 12x + 16 \leq 0\)
Řešení
a)   \((- \infty, -1) \cup (5, \infty)\)
b)   \(\langle -5, 2 \rangle\)
c)   \((- \infty, 0 \rangle \cup \langle 4, \infty)\)
d)   \(x \in (-\infty, -2) \cup (7, \infty)\)
e)   \((- \infty, 3) \cup (3, \infty)\)
f)   \(\langle 2, 4 \rangle\)

4. Uložené peníze

Petr si založil spořicí účet, na který vložil 50 000 Kč. Banka nabízí roční úrokovou sazbu 3 % s ročním připisováním úroků (složené úročení). Peníze nechá na účtu 5 let.

Jakou částku bude mít Petr na účtu, pokud nebude vkládat ani vybírat peníze?
Řešení
Po 5 letech bude mít Petr na účtu přibližně 57 963,70 Kč.

5. Goniometrické rovnice 1

Vyřešte goniometrické rovnice:
a)   \[\sin x = \frac{1}{2}\]
b)   \[\cos x = -\frac{\sqrt{2}}{2}\]
c)   \[\tan x = \sqrt{3}\]
d)   \[\sin^2 x = \frac{3}{4}\]
e)   \[\cos 2x = 0\]
f)   \[\tan^2 x = 3\]
Řešení
a)   \[x = \frac{\pi}{6} + 2k\pi \lor x = \frac{5\pi}{6} + 2k\pi, k \in \mathbb{Z}\]
b)   \[x = \frac{3\pi}{4} + 2k\pi \lor x = \frac{5\pi}{4} + 2k\pi, k \in \mathbb{Z}\]
c)   \[x = \frac{\pi}{3} + k\pi, k \in \mathbb{Z}\]
d)   \[x = \frac{\pi}{3} + 2k\pi \lor x = \frac{2\pi}{3} + 2k\pi \lor x = \frac{4\pi}{3} + 2k\pi \lor x = \frac{5\pi}{3} + 2k\pi, k \in \mathbb{Z}\]
e)   \[x = \frac{\pi}{4} + \frac{k\pi}{2}, k \in \mathbb{Z}\]
f)   \[x = \frac{\pi}{3} + k\pi \lor x = -\frac{\pi}{3} + k\pi, k \in \mathbb{Z}\]

6. Koupě auta

Petr si chce za dva roky koupit nové auto. Ví, že cena auta bude 600 000 Kč. Plánuje si peníze odkládat na spořicí účet s ročním úrokem 4 %, který se připisuje na konci každého roku.

Vypočítejte, kolik musí Petr vložit na tento účet dnes, aby měl za dva roky dostatek peněz na nákup auta.
Řešení
Petr musí vložit na účet částku 554 705 Kč.

7. Plnění zásobníku vodou

Zásobník na vodu má tvar válce o poloměru základny 50 cm a výšce ( frac{3}{pi} , ext{m} ). Aktuálně je naplněn ze 40 %. Do zásobníku začala téct voda rychlostí 1 litr za 2 sekundy.

Vypočítejte, za jak dlouho bude zásobník naplněn z 90 %. (Zapište v minutách a sekundách.)
Řešení
Zásobník bude naplněn z 90 % za 12 minut 30 sekund.

8. Úhly v trojúhelníku

Určete velikosti všech úhlů v trojúhelníku, který je zadán souřadnicemi tří bodů. Výsledky zapište ve stupních na dvě desetinná místa.
a)   \( A(1, 2), \quad B(4, 6), \quad C(7, 2). \)
b)   \( A(-2, 1), \quad B(3, 4), \quad C(1, -3). \)
c)   \( A(2, 3), \quad B(6, 3), \quad C(6, 7). \)
d)   \( A(1, 1), \quad B(4, 5), \quad C(7, 2). \)
Řešení
a)   \[ \alpha \approx 53.13^\circ, \quad \beta \approx 73.74^\circ, \quad \gamma \approx 53.13^\circ.\]
b)   \[ \alpha \approx 79.1^\circ, \quad \beta \approx 50.9^\circ, \quad \gamma \approx 50^\circ. \]
c)   \[ \alpha = 45^\circ, \quad \beta = 45^\circ, \quad \gamma = 90^\circ. \]
d)   \[ \alpha \approx 48.6^\circ, \quad \beta \approx 60^\circ, \quad \gamma \approx 71.4^\circ. \]

9. Průsečík dvou rovin

Najděte průsečík rovin:
a)   \[ \text{Rovina } \rho_1 \text{: } x = 2 + s, \quad y = s + t, \quad z = 3 + t, \quad s, t \in \mathbb{R}.\] \[ \text{Rovina } \rho_2 \text{: } x = 4 + u, \quad y = 2u - v, \quad z = 5 - v, \quad u, v \in \mathbb{R}. \]
b)   \[ \text{Rovina } \rho_1 \text{: } x = s, \quad y = 2 + s + t, \quad z = 3 + t, \quad s, t \in \mathbb{R}. \] \[ \text{Rovina } \rho_2 \text{: } x = u + v, \quad y = 4 - u, \quad z = 6 + v, \quad u, v \in \mathbb{R}. \]
c)   \[ \text{Rovina } \rho_1 \text{: } x = 3 + s, \quad y = -s, \quad z = 2 - t, \quad s, t \in \mathbb{R}. \] \[ \text{Rovina } \rho_2 \text{: } x = 3 + 2u - v, \quad y = u - 2v, \quad z = 2 + u + v, \quad u, v \in \mathbb{R}. \]
d)   \[ \text{Rovina } \rho_1 \text{: } x = 1 + s, \quad y = 1 + t, \quad z = 1 + t, \quad s, t \in \mathbb{R}.\] \[ \text{Rovina } \rho_2 \text{: } x = 2 + u, \quad y = 2 - v, \quad z = 2 - v, \quad u, v \in \mathbb{R}. \]
Řešení
a)   \[ x = 4, \quad y = 8 - v, \quad z = 5 - v, \quad v \in \mathbb{R}. \]
b)   \[ x = -\frac{1}{2} + v, \quad y = \frac{9}{2} + v, \quad z = 6 + v, \quad v \in \mathbb{R}. \]
c)   \[ x = 3 + v, \quad y = -v, \quad z = 2 + 2v, \quad v \in \mathbb{R}. \]
d)   \[ x = 1 + v, \quad y = 2 - v, \quad z = 2 - v, \quad v \in \mathbb{R}. \]

10. Světelné efekty ve městě

Ve městě se plánuje oslavná událost, na kterou organizátoři připravili speciální světelné show. K dispozici mají 4 různé barvy světel: červenou, modrou, zelenou a žlutou. Každou barvu mohou použít vícekrát a pořadí barev při show je důležité.

Vypočítejte, kolik různých sekvencí světel o délce 5 mohou organizátoři vytvořit.
Řešení
Organizátoři mohou vytvořit 1 024 různých sekvencí světel.

11. Limity funkcí

Pomocí l'Hospitalova pravidla vypočítejte limity funkcí:
a)   \[\lim_{x \to \infty} \frac{x}{e^x}\]
b)   \[\lim_{x \to 0^+} \frac{\ln(x)}{\frac{1}{x}}\]
c)   \[\lim_{x \to 0} \frac{\sin(x)}{x^2}\]
d)   \[\lim_{x \to 1} \frac{x^2 - 1}{x - 1}\]
e)   \[\lim_{x \to \infty} \frac{\ln(x)}{\sqrt{x}}\]
f)   \[\lim_{x \to 0} \frac{\tan(x)}{x}\]
Řešení
a)   0
b)   0
c)   0
d)   2
e)   0
f)   1

12. Derivace funkcí

Vypočítejte derivace následujících funkcí:
a)   \[ f(x) = x^2 \cdot e^x \]
b)   \[ g(x) = \frac{x^2 + 3}{x - 1} \]
c)   \[ h(x) = \sin(x) \cdot \cos(x) \]
d)   \[ k(x) = \sqrt{x^2 + 1} \]
e)   \[ m(x) = e^{\sin(x)} \]
f)   \[ p(x) = \ln(x^2 + 1) \]
Řešení
a)   \[ f'(x) = e^x (2x + x^2) \]
b)   \[g'(x) = \frac{x^2 - 2x - 3}{(x - 1)^2}\]
c)   \[h'(x) = \cos(2x)\]
d)   \[k'(x) = \frac{x}{\sqrt{x^2 + 1}}\]
e)   \[m'(x) = e^{\sin(x)} \cos(x)\]
f)   \[p'(x) = \frac{2x}{x^2 + 1}\]

13. Sarrusovo pravidlo

Vypočítejte determinant pomocí Sarrusova pravidla:
a)   \[ \begin{vmatrix} 1 & 2 & 3 \\ 4 & 5 & 6 \\ 7 & 8 & 9 \end{vmatrix} \]
b)   \[ \begin{vmatrix} 2 & 1 & 3 \\ 1 & 4 & 2 \\3 & 5 & 6 \end{vmatrix}\]
c)   \[ \begin{vmatrix} 3 & 0 & 1 \\ 2 & 4 & 5 \\ 1 & 3 & 2 \end{vmatrix}\]
d)   \[ \begin{vmatrix}1 & 3 & 5 \\2 & 1 & 4 \\3 & 2 & 1\end{vmatrix}\]
e)   \[ \begin{vmatrix} 5 & 2 & 0 \\ 1 & 3 & 2 \\ 4 & 1 & 3\end{vmatrix}\]
f)   \[\begin{vmatrix}1 & 2 & 3 \\ 0 & 1 & 4 \\ 5 & 6 & 0 \end{vmatrix}\]
Řešení
a)   \( 0 \)
b)   \( 7 \)
c)   \( -19 \)
d)   \( 28 \)
e)   \( 45 \)
f)   \( 1 \)

14. Limity posloupností 2

Vypočítejte následující limity:
a)   \[\lim_{n \to \infty} \frac{3n^2 + 5}{n^2 + 4n + 1}\]
b)   \[\lim_{n \to \infty} \frac{n + \ln n}{n + 1}\]
c)   \[\lim_{n \to \infty} \frac{\cos n}{n^2}\]
d)   \[\lim_{n \to \infty} \frac{n^3}{n^2 + 1}\]
e)   \[\lim_{n \to \infty} \left(1 - \frac{2}{n}\right)^n\]
f)   \[\lim_{n \to \infty} n\sin\left(\frac{1}{n}\right)\]
Řešení
a)   3
b)   1
c)   0
d)   \( \infty \)
e)   \( e^{-2} \)
f)   1

15. Limity posloupností 1

Vypočítejte následující limity:
a)   \[\lim_{n \to \infty} \frac{n}{n+1}\]
b)   \[\lim_{n \to \infty} \frac{2n^2 + 3n}{n^2 + n}\]
c)   \[\lim_{n \to \infty} \left(1 + \frac{1}{n}\right)^n\]
d)   \[\lim_{n \to \infty} \frac{\sin n}{n}\]
e)   \[\lim_{n \to \infty} \frac{\sqrt{n^2 + 1}}{n+2}\]
f)   \[\lim_{n \to \infty} \frac{n^2 + n + 1}{n^3 + 2n^2 + 3}\]
Řešení
a)   1
b)   2
c)   e
d)   0
e)   1
f)   0

16. Logaritmické rovnice

Vyřešte v R logaritmické rovnice:
a)   \( \log_3\left(5 + \log_2((x-1)^4)\right) = 2 \)
b)   \( \log_2(x) + \log_2(x - 1) = 3 \)
c)   \( \log_3(x^2 + 3x) - \log_3(x) = 1 \)
d)   \( \log_5(x^2 - 4) + \log_5(2x) = 2 \)
e)   \( \ln(x^2 + 2x) - \ln(x + 1) = \ln(4) \)
f)   \( \log_{10}(x^2 + 4x + 4) + \log_{10}(x + 3) = 2 \)
Řešení
a)   \( x = 3 \quad \text{a} \quad x = -1 \)
b)   \( x = 4 \)
c)   \( \text{Žádné řešení.} \)
d)   \( x = 3 \)
e)   \( x = 2 \)
f)   \( x = 2 \)

17. Logaritmické rovnice

Vyřeš logaritmické rovnice:
a)   \( \log_2(x+1) + \log_2(x-1) = 3 \)
b)   \( \log_2(2x+3) - \log_2(x-1) = 1 \)
c)   \( \log_2(x+4) + \log_2(x-2) = 3 \)
d)   \( \log_2(x+4) + \log_2(x-2) = 3 \)
e)   \( \log_2(4x+1) - \log_2(x-1) = 3 \)
f)   \( \log(99x+100) - \log(x-1) = 2 \)
Řešení
a)   \[ x = 3 \]
b)   \[ \text{nemá řešení} \]
c)   \[ x = \frac{1 + \sqrt{41}}{2} \]
d)   \[ x = -1 + \sqrt{17} \]
e)   \[ x = \frac{9}{4} \]
f)   \[ x = 200 \]

18. Determinant matice

Spočítejte determinant matice:
a)   \[ A = \begin{pmatrix} 2 & 3 & 1 \\ 4 & 1 & -2 \\ 3 & 0 & 5 \end{pmatrix} \]
b)   \[ B = \begin{pmatrix} 1 & 2 & 3 \\ 0 & 1 & 4 \\ 5 & 0 & 1 \end{pmatrix} \]
Řešení
a)   \( \text{det}(A) = -71 \)
b)   \( \text{det}(B) = 26 \)

19. Soustavy rovnic Gaussovou eliminací

Pomocí Gaussovy eliminace řešte soustavy rovnic:
a)   \[ \begin{pmatrix} 1 & 2 & -1 \\ 2 & 1 & 3 \\ 3 & -1 & 2 \end{pmatrix} \begin{pmatrix} x_1 \\ x_2 \\ x_3 \end{pmatrix} = \begin{pmatrix} 2 \\ 5 \\ 4 \end{pmatrix} \]
b)   \[ \begin{pmatrix} 2 & 3 & 1 \\ 4 & 7 & 3 \\ 6 & 9 & 5 \end{pmatrix} \begin{pmatrix} x_1 \\ x_2 \\ x_3 \end{pmatrix} = \begin{pmatrix} 1 \\ 3 \\ 5 \end{pmatrix} \]
c)   \[ \begin{pmatrix} 1 & -1 & 2 \\ 3 & 2 & 1 \\ 2 & 1 & -1 \end{pmatrix} \begin{pmatrix} x_1 \\ x_2 \\ x_3 \end{pmatrix} = \begin{pmatrix} 4 \\ 7 \\ 1 \end{pmatrix} \]
d)   \[ \begin{pmatrix} 1 & 1 & 1 \\ 2 & 2 & 2 \\ 3 & 3 & 3 \end{pmatrix} \begin{pmatrix} x_1 \\ x_2 \\ x_3 \end{pmatrix} = \begin{pmatrix} 6 \\ 12 \\ 15 \end{pmatrix} \]
Řešení
a)   \( x_1 = 1 \), \( x_2 = -1 \), \( x_3 = 1 \)
b)   \( x_1 = -1 \), \( x_2 = 0 \), \( x_3 = 1 \)
c)   \( x_1 = 1 \), \( x_2 = 1 \), \( x_3 = 2 \)
d)   Nemá řešení.

20. Derivace složených funkcí

Určete derivace následujících funkcí:
a)   \( f_1(x) = \sin(3x^2) \)
b)   \( f_2(x) = \ln(\cos(x^2)) \)
c)   \( f_3(x) = e^{x^3} \)
d)   \( f_4(x) = \sqrt{2x^5 + 1} \)
e)   \( f_5(x) = \tan(\ln(x)) \)
f)   \( f_6(x) = \frac{1}{x^2 + e^{2x}} \)
g)   \( f_7(x) = \sin(\sqrt{x}) \)
h)   \( f_8(x) = \frac{\ln(x)}{x^3} \)
Řešení
a)   \[ f_1'(x) = \cos(3x^2) \cdot 6x \]
b)   \[ f_2'(x) = -2x \cdot \tan(x^2)\]
c)   \[ f_3'(x) = e^{x^3} \cdot 3x^2 \]
d)   \[ f_4'(x) = \frac{5x^4}{\sqrt{2x^5 + 1}} \]
e)   \[ f_5'(x) = \frac{1}{x \cdot \cos^2(\ln(x))} \]
f)   \[ f_6'(x) = -\frac{2x + 2e^{2x}}{(x^2 + e^{2x})^2} \]
g)   \[ f_7'(x) = \frac{\cos(\sqrt{x})}{2\sqrt{x}} \]
h)   \[ f_8'(x) = \frac{1 - 3\ln(x)}{x^4} \]
 
12345